Bit Error Ratio
In the pursuit of ever-greater data transmission capabilities, forward error correction (FEC) has emerged as a pivotal technology, not just...
-
Free
-
March 26, 2025
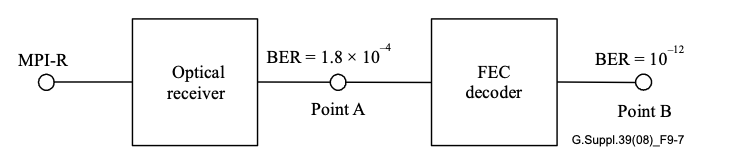
Forward Error Correction (FEC) has become an indispensable tool in modern optical communication, enhancing signal integrity and extending transmission distances....
-
Free
-
March 26, 2025
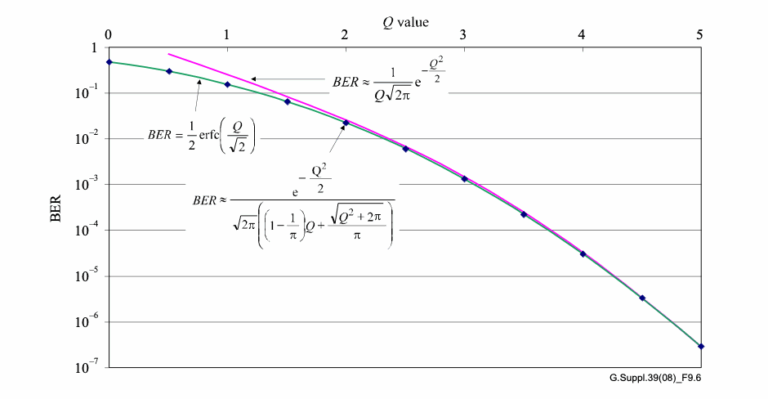
Signal integrity is the cornerstone of effective fiber optic communication. In this sphere, two metrics stand paramount: Bit Error Ratio...
-
Free
-
March 26, 2025
In the world of optical communication, it is crucial to have a clear understanding of Bit Error Rate (BER). This...
-
Free
-
March 26, 2025
Explore Articles
- Analysis
- Automation
- Careers and Learning Paths
- Coherent Optics
- Data Center Interconnect
- Free
- Fundamentals
- Management
- Network Architecture
- Planning & Design
- Premium
- Security
- Standards
- Submarine and Long-Haul
- Technical
- Testing
- Tools and Simulators
- Trends & News
- Troubleshooting and Operations
- Vendor and Product Landscape
Filter Articles
ResetTags
automation
ber
Chromatic Dispersion
Coherent detection
coherent optical transmission
coherent optics
Data transmission
distributed Raman amplification
DWDM
edfa
EDFA noise figure
fec
Fiber optics
Fiber optic technology
Forward Error Correction
Latency
modulation
network automation
noise figure
optical
Optical communication
Optical fiber
Optical network
optical network automation
Optical network design
optical networking
Optical networks
Optical signal-to-noise ratio
OSNR
OSNR calculation
OTN
Probabilistic Constellation Shaping
Q-factor
raman
Raman amplification
Raman Amplifier
ROADM
Signal integrity
Signal quality
Slider
spectral efficiency
submarine
submarine cable systems
Telecommunications
Ticker