Standards
Showing 21 - 30 of 212 results
Erbium-Doped Fiber Amplifier Fundamentals: Complete Technical Guide Erbium-Doped Fiber Amplifier Fundamentals Complete Technical Guide from Basic Principles to Advanced Applications...
-
Free
-
August 9, 2025
Font Size: A- A A+... Membership Required You must be a member to access this content.View Membership LevelsAlready a member?...
-
Free
-
July 30, 2025
Font Size: A- A A+... Membership Required You must be a member to access this content.View Membership LevelsAlready a member?...
-
Free
-
July 30, 2025
Font Size: A- A A+... Membership Required You must be a member to access this content.View Membership LevelsAlready a member?...
-
Free
-
July 29, 2025
Font Size: A- A A+... Membership Required You must be a member to access this content.View Membership LevelsAlready a member?...
-
Free
-
July 29, 2025
... Membership Required You must be a member to access this content.View Membership LevelsAlready a member? Log in here
-
Free
-
July 29, 2025
... Membership Required You must be a member to access this content.View Membership LevelsAlready a member? Log in here
-
Free
-
July 26, 2025
... Membership Required You must be a member to access this content.View Membership LevelsAlready a member? Log in here
-
Free
-
July 25, 2025
Font Size: A- A A+... Membership Required You must be a member to access this content.View Membership LevelsAlready a member?...
-
Free
-
July 25, 2025
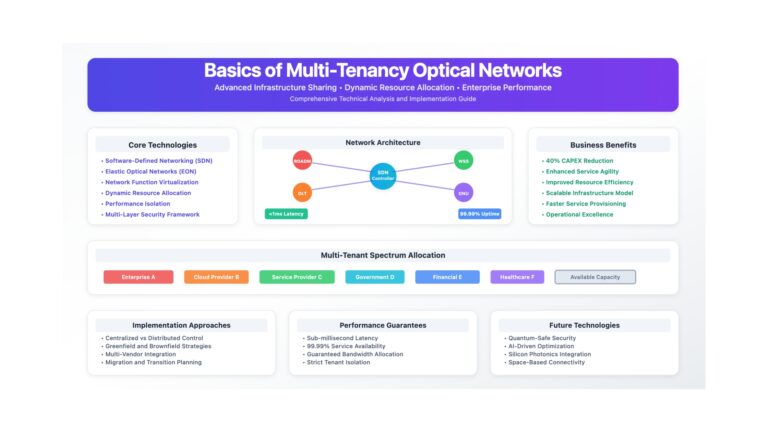
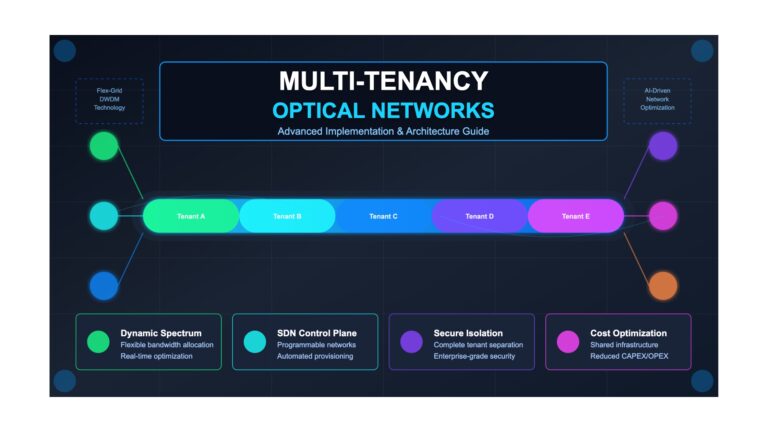
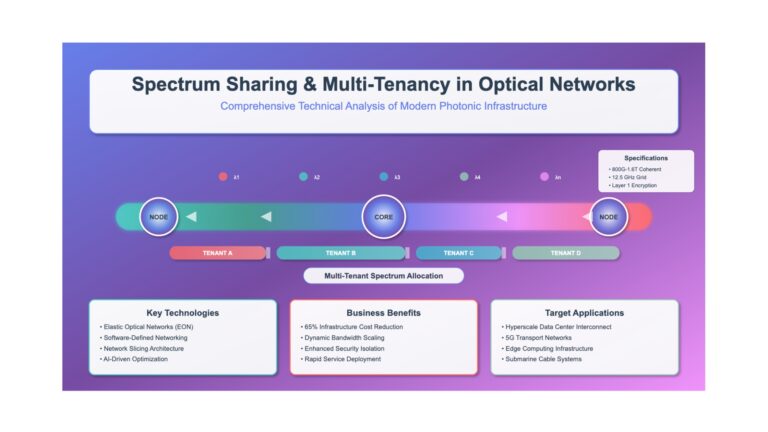
Spectrum Sharing and Multi-Tenancy in Optical Networks: A Comprehensive Technical Analysis Spectrum Sharing and Multi-Tenancy in Optical Networks A Comprehensive...
-
Free
-
July 25, 2025
Explore Articles
Filter Articles
ResetExplore Courses
Tags
amplifier
automation
ber
Bit Error Ratio
Chromatic Dispersion
Communication technology
Data transmission
DWDM
edfa
EDFAs
Erbium-Doped Fiber Amplifiers
Error correction
fec
Fiber optics
Fiber optic technology
Forward Error Correction
Latency
modulation
network automation
Network Design
network management
Network performance
noise figure
optical
optical amplifiers
Optical communication
Optical fiber
Optical network
optical networking
Optical networks
Optical signal-to-noise ratio
Optical transmission
OSNR
OTN
Q-factor
raman
Raman Amplifier
SDH
Signal amplification
Signal distortion
Signal integrity
Signal quality
Slider
Telecommunications
Ticker